Question

Please help, teacher didn’t teach us this

Answer 1

x-intercept: -60; y-intercept: 42

Explanation:

The equation can be simplified to

`-(1)/(2)x+(5)/(7)y=30`

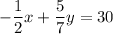
Dividing by 30 can help you put the equation in "intercept form" in which the intercepts are in the denominators.

`-(1)/(2\cdot 30)x+(5)/(7\cdot 30)y=1\\\\(x)/(-60)+(y)/(42)=1`

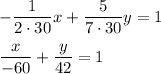
In general, you find the x-intercept by setting y=0, and the y-intercept by setting x=0. When you do that with the equation in this form, you see that the respective denominators are the intercepts. That is, the numerator and denominator of each of the fractions must be equal when the other fraction is multiplied by zero.

For x-intercept, y = 0:

`(x)/(-60)+0=1\\\\x=-60`

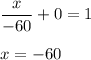
For y-intercept, x=0:

`0+(y)/(42)=1\\\\y=42`

_____

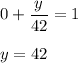
I find the intercept form useful for determining both intercepts at once. All that is really necessary for finding one intercept is to set the other variable to zero, then solve. This is true for whatever form the equation may be in. And it is true for equations of any degree or complication. (This may be obvious to you from the fact that the coordinates of the x-intercept have a y-value of 0, and vice-versa.)