We are given function: h(x)=-5(x-4)^2+180 for the height in meters, x seconds after the launch.
We need to find the time when it hit the ground.
The height of the object would be 0, when it hit the ground.
So, we need to set the given function equal to 0 and solve for x.
-5(x-4)^2+180 = 0.
Subtracting both sides by 180.
-5(x-4)^2+180-180 = 0-180.
-5(x-4)^2 = -180.
Dividing both sides by -5, we get
(x-4)^2 = 36.
Taking square root on both sides, we get
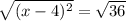
x-4 = + - 6
x-4 =6 and x-4 =-6
Adding 4 on both sides in both equations, we get
x-4+4 =6+4 and x-4+6 =-6+4
x=10 and x=-2.
x represents time in seconds. So we can't take a negative value for time.
Therefore, 10 seconds after being launched will the object hit the ground.