Question

What transformation takes the graph of f(x)=3x+8 to the graph of g(x)=3x+6 ?

translation 2 units left

translation 2 units right

translation 2 units up

translation 2 units down

Answer 1

Translation 2 units downward

Explanation:

If we start with the graph of f(x) = 3x + 8 and translate it 2 units downward, we'll end up with the graph of g(x) = 3x + 6. Note: 8 - 2 = 6.

Answer 2

D. Translation 2 units down.

Explanation:

We have been given a transformation rule and we are asked to find the transformation rule.

Let us recall the transformation rule.

`f(x)\rightarrow f(x-a)=\text{Graph shifted to right by a units}`

`f(x)\rightarrow f(x+a)=\text{Graph shifted to left by a units}`

`f(x)\rightarrow f(x)-a=\text{Graph shifted downwards by a units}`

`f(x)\rightarrow f(x)+a=\text{Graph shifted upwards by a units}`

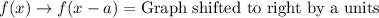
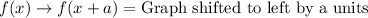
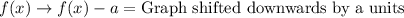
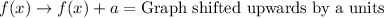
Upon looking at our given transformation, we can see that
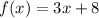`f(x)=3x+8` is shifted downwards by 2 units to get graph of
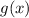`g(x)` as:

`g(x)=(3x)+8-2`

`g(x)=3x+6`

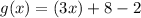
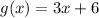
Therefore, option D is the correct choice.