Question

A light private plane can fly 120 mph in still air. Flying against the wind, the plane can fly 320 miles in the same time it requires to fly 640 miles with the wind. Find the rate of the wind.

A) 120 mph
B) 80 mph
C) 40 mph

Answer 1

40

Explanation:

Answer 2

Alright, lets get started.

A light private plane can fly 120 mph in still air.

Suppose the speed of wind = x mph

Against the wind, speed of plane will be = 120-x

With the wind, speed of plane will be = 120+x

Flying against the wind, the plane can fly 320 miles, so time taken will be

t =
`(320)/(120-x)`

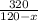
With the wind, the plane can fly 640 miles, so time taken will be

`t = (640)/(120+x)`

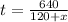
Both times are given same, so

`(320)/(120-x)= (640)/(120+x)`

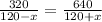
Cross multiplying

`320(120+x) = 640(120-x)`

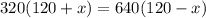
Dividing from 320

`120+x = 2(120-x)`

`120+x = 240-2x`

`3x = 120`

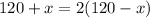
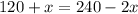
x = 40 mph

So, the speed of the wind is 40 mph. : Answer

Hope it will help :)