Answer:
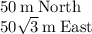
Step-by-step explanation:
We can create a 30-60-90 triangle. The distance she walked is then the hypotenuse of the triangle, and using 30-60-90 triangle rules, we have the following:
The North leg is opposite to the
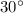 angle. Therefore, if we call this distance
angle. Therefore, if we call this distance
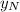 , we have the following:
, we have the following:
 .
.
The East leg is opposite to the
 angle. If we call this distance
angle. If we call this distance
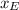 , we have:
, we have:
 .
.