Question

find the distance between each pair of points. if necessary round to the nearest tenth j(2,-1),k(2,5)

Answer 1

`\bf ~~~~~~~~~~~~\textit{distance between 2 points}\\\\J(\stackrel{x_1}{2}~,~\stackrel{y_1}{-1})\qquadK(\stackrel{x_2}{2}~,~\stackrel{y_2}{5})\qquad \qquadd = √(( x_2- x_1)^2 + ( y_2- y_1)^2)\\\\\\JK=√([2-2]^2+[5-(-1)]^2)\implies JK=√((2-2)^2+(5+1)^2)\\\\\\JK=√(0+6^2)\implies JK=6`

Answer 2

Distance between j and k = 6 units

Explanation:

Following formula is used to find the distance between two points:

Distance =
`\sqrt{(x_(1)  - x_(2) )^(2) + (y_(1) - y_(2) )^(2)`

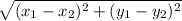
We are given two points j (2,-1) and k (2,5)

so putting in the values for
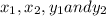`x_(1) , x_(2) , y_(1) and y_(2)`, we get:

`\sqrt{(2 - 2)^(2)  + (- 1 - 5)^(2) }`

=
`(\sqrt{( 0 )^(2) + (-6)^2}`

=
`√(36)`

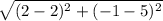
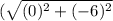
= 6

Therefore, the distance between the two points j and k is 6 units.