Question

Use the premises and conclusion to answer the questions.

Premises:

If a triangle has an angle with a measure more than 90°, then the triangle is an obtuse triangle.

The measure of an angle in △ABC is 110°.

Conclusion:

△ABC is an obtuse triangle.



Is the argument valid? Why or why not?


The argument is valid by the law of syllogism.

The argument is not valid because the premises are not true.

The argument is valid by the law of detachment.

The argument is not valid because the conclusion does not follow from the premises.

Answer 1

The given argument is valid by the law of detachment

Explanation:

Law of Detachment states that if the following two statements are true:

(1) If a , then b .

(2) a

Then we can say a third true statement:

(3) b.

Given:

1) If a triangle has an angle with a measure more than 90°, then the triangle is an obtuse triangle.

2) The measure of an angle in △ABC is 110°.

Let the statement a be "If a triangle has an angle with a measure more than 90°" , and b be the statement "the triangle is an obtuse triangle."

then, (1) and (2) can be written as:

1) If a , then b.

2) a

so, by the Law of Detachment, we can say that b is true i.e,

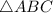`\triangle ABC` is an obtuse triangle.