Answer:
K(-4,8) is the ortho center.
Explanation:
In a right angled triangle, The vertex of the right angle is the ortho center.
Here we are given
J(-4,-1), K(-4,8) & L(2,8)
Using distance formula we get
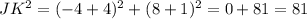
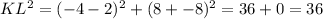
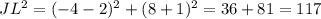
So we can say that
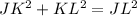
By converse of pythagorean theorem we get
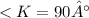
Hence the Vertex of the right angle is K(-4,8)
K(-4,8) is the ortho center.