Question

Find the value of x. Round your answer to
the nearest tenth. Please help

Answer 1

x=50.7°

Explanation:

Use the given angle of x° to find which trigonometry ratio to use. We are given the side adjacent to the angle and the hypotenuse. Therefore, we use the cosine ratio:

`cosineX=(adjacent)/(hypotenuse)`

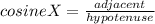
Insert the given values:

`cos.x=(19)/(30)`

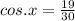
In order to fin the angle, use the inverse:

`x=cos^(-1)((19)/(30) )`

Insert the equation into a calculator:

`x=50.70351976`

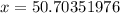
Round to the nearest tenth:

`x=50.7`

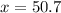
:Done