Answer:
Sides of triangle = 3 , 4 and 5
Step-by-step explanation:
We have a - b = 1 --------------Equation 1
Perimeter = 12
a + b + c = 12 --------------Equation 2
And we have by Pythagoras theorem, c² = a² + b² --------------Equation 3
From equation 2 we have c = 12 -a -b, substituting in equation 3
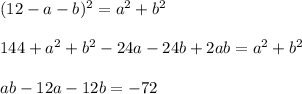 --------------Equation 4
--------------Equation 4
From equation 1 we have a = 1+b, substituting in equation 4
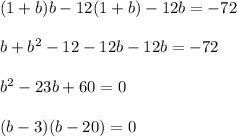
b = 20 is not possible since perimeter is only 12.
So, b = 3
Substituting in equation 1 and 2
a - 3 = 1, a = 4
3 + 4 + c = 12, c= 5
Sides of triangle = 3 , 4 and 5