Question

The distance d in feet that a dropped object falls in t seconds is given by the equation d÷16=t2. How long does it take a dropped object to fall 64 feet

Answer 1

aight so you have to solve for t
first you plug in for d

`(64) / 16 = t2`
solve this

`4 = t2`
now you must divide by two to get t by itself

`(4)/(2) = (t2)/(2)`
solve this and your solution is

`t = 2`
or 2 seconds

Answer 2

It takes 2 seconds.

Explanation:

Consider the provided equation.

`d/ 16=t^2`

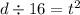
The provided equation can be written as

`(d)/(16)=t^2`

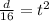
We need to calculate how long does it take a dropped object to fall 64 feet.

Substitute the d=64 in above equation and solve the equation for t.

`\frac{64}16}=t^2`

`4=t^2`

`t=\pm2`

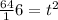
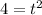
Here, time should be positive so ignore the negative value of t.

`t=2`

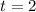
Hence, it takes 2 seconds.