We are given
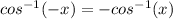
Firstly, we will simplify left side
and we will check whether it is equal to right side
Left side:
Let's assume y left side expression
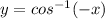
now, we can take cos on both sides
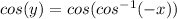
we can simplify it
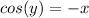
Right side:
Let's assume y left side expression
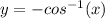
Multiply both sides by -1

now, we can take cos on both sides
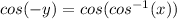
we can simplify it
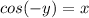
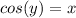
we can see that
both sides cos(y) value is different
so, this is FALSE.........Answer