Question

A friction force of 280 N exists between a cart and the path. If the force of reaction is 766 N, what is the minimum action force needed to set the cart in motion?

Answer 1

force of friction on the cart is given as

`F_f = 280 N`

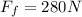
here we also know the reaction force due to surface

`R = 766 N`

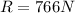
so we can say reaction force is given as

`R = √(F_n^2 + F_f^2)`

`766 = √(F_n^2 + 280^2)`

`F_n^2 = 766^2 - 280^2`

`F_n = 713 N`

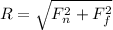
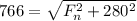
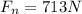
now by force balance we will say

`F_y + F_n = mg`

`F_y = mg - F_n`

`F_x = F_f`

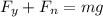
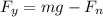
also we know that

`F_f = \mu * F_n`

`280 = \mu * 713`

`\mu = 0.39`

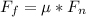
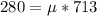
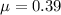
now minimum force required to set this into motion

`F_(min) = (\mu mg)/(√(1 + \mu^2))`

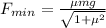
here we know that

`mg = F_n = 713 N`

`F_(min) = (0.39* 713)/(√(1 + 0.39^2))`

`F_(min) = 259 N`

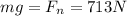
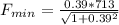
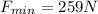
So it will require 259 N minimum force to move it