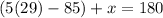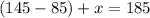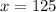Question

Help i need to understand

Answer 1

using thereoms/postulates, we know that the 60° angle and the (5z - 85)° angle are congruent

so to first find z..

`60 = 5z - 85`

`145 = 5z`

`29 = z`

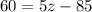
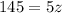
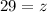
now that we know z, we can find x
simply replace the z in (5z - 85) with 29
and add the entire angle to x to equal 180
(supplementary angles)

`(5(29) - 85) + x = 180`

`(145 - 85) + x = 185`

`60 + x = 185`

`x = 125`



`x = 125 \\ z = 29`