Answer:
The Probability that Event A and Event D occur is equal to the probability Event A occurs times the probability that Event D occurs, given that A has occurred.
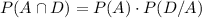
We can find the values of
 and
and
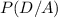 using the above form formula.
using the above form formula.
 ;
;
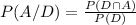
From the given table, we have the values of P(A), P(D),
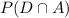 and
and
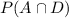 .
.
Since, Probability=

∴
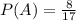 ,
,
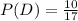 ,
,
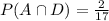 and
and
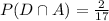
Now, putting these values in above formula we get,
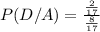

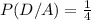 .
.

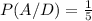
As, you can see above that the values of P(A|D) and P(D|A) are not equal.