Answer:
The value that optimizes this inequality is x = 1, since it represents the vertex of the parabola. Therefore, $ 0.25 is the price that must be discounted to maximize revenues
With x = 1 the value of the new income will be = $ 1983.75
Explanation:
Revenue with the current sale price is:
6 * 330 = $ 1980
Let's call x the number of times the price of a sandwich decreases, that is, if x = 1 then the price of the sandwich decreases by $ 0.25
Then, the price would be:
Price = (6-0.25x)
Then, if the price is decreased by a factor of x, then the number of sandwiches sold will increase by a factor of 15x. Therefore the number of clients will be:
Sale = (330 + 15x)
Now we need an equation for income. The income will be equal to the sale price, for the number of sandwiches sold
Income = price * sale
Income = (6-0.25x) (330 + 15x)
In order for this restaurant pricing model to be profitable, the revenues with the new sale price must be greater than the revenues with the sale price of $ 6. So:
New income> current income.

The left side of the inequality represents a parabola:
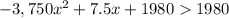
The value that optimizes this inequality is x = 1, since it represents the vertex of the parabola. Therefore, $ 0.25 is the price that must be discounted to maximize revenues
The attached image shows the region that satisfies the proposed inequality and the vertex of the parabola:
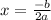
With x = 1 the value of the new income will be = $ 1983.75