Question

Line h passes through point (3,0) and is perpendicular to the graph of y=1/5x-19. Line g is parallel to line h and passes through point (-1.15). What is the equation in slope intercept form of line g?

Answer 1

-5x + 10

Explanation:

If a line 2 is perpendicular to line 1 and line 1 has slope m, then line 2 must have slope =
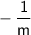`\sf -(1)/(m)` which is the negative of the reciprocal of m

Essentially the product of the two slopes = -1

The line

`y = (1)/(5)x - 19`
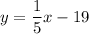
has slope of
`(1)/(5)` so the slope of line h which is perpendicular to this line must have slope = negative of reciprocal of
`(1)/(5)`

Reciprocal of
`(1)/(5)` is 5
Negative of reciprocal is thus -5

Equation of h is of the form
y = -5x + b where b is the y intercept, the value of y at x = 0

Since line h passes through (3, 0) we plug in these values for x and y and solve for b
0 = -5(3) + b

0 = -15 + b
15 = b (Adding 15 on both sides)
b = 15

Equation of line h is y = -5x + 15

Line g is parallel to line h. Parallel lines have the same slope so the equation for line g is
y = -5x + b (since slope of g = slope of h = -5)

Since this line passes through x = -1, y = 15 we can solve for b as before by plugging these values into the equation

15 = -5(-1) + b
15 = 5 + b
10 = b (Subtracting 5 on both sides)
b = 10

So equation of line g = -5x + 10

Answer 2

y = -5x + 10

Explanation: