Question

Taxi A charges $0.20 per mile and an initial fee of $4. Taxi B charges $0.40 per mile and an initial fee of $2. Write an inequality that can determine when the cost of Taxi B will be greater than Taxi A. A) 0.20x + 4 > 0.40x + 2 B) 0.20x + 4 < 0.40x + 2 C) 0.20x + 0.40x > 4 + 2 D) 0.20x + 0.40x < 4 + 2

Answer 1

The expression for the cost of Taxi B is 0.40x + 2. The expression for the cost of Taxi A is 0.20x + 4. The inequality asks for when the cost of Taxi B will be greater than Taxi A, so you write it as 0.40x + 2 > 0.20x + 4, or 0.20x + 4 < 0.40x + 2.

The answer is B) 0.20x + 4 < 0.40x + 2.

Answer 2

Answer: B.
`0.20x+4<0.40x+2`

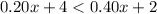
Explanation:

let x denotes the number of mile taxi runs.

Given : Taxi A charges $0.20 per mile and an initial fee of $4.

i.e. the expression to show total charge by Taxi A will be :
`0.20x+4`

Taxi B charges $0.40 per mile and an initial fee of $2.

i.e. the expression to show total charge by Taxi B will be :
`0.40x+2`

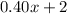
Now, the inequality that can determine when the cost of Taxi B will be greater than Taxi A will be :-

`0.20x+4<0.40x+2`

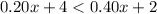
Hence, B is the correct answer.