The product of (3 + 2i) and a complex number is (17 + 7i)
The product of (3 + 2i) and a complex number is (17 + 7i).
Let the complex number be a + ib
Product means we multiply
So (3+2i) * (a + ib) = (17+7i)
WE need to find a+ ib
Divie by 3 + 2i on both sides
a + ib = (17+7i) / (3+2i)
To divide multiply by the conjugate (3-2i)
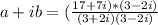

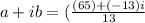
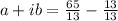
a+ib = 5 - i
The required complex number is 5 - i