solution is (x, y ) = (10, 10)
given
x + y = 20 → (1)
30x + 25y = 550 → (2)
from (1) y = 20 - x → (3)
substitute y = 20 - x in (2)
30x + 25(20 - x) = 550
30x + 500 - 25x = 550
5x + 500 = 550 ( subtract 500 from both sides )
5x = 50 ( divide both sides by 5 )
x =
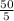 = 10
= 10
substitute x = 10 in (3) : y = 20 - 10 = 10
thus x = 10, y = 10 is the solution