One half a number increased by three is greater than zero or less than or equal to negative three

Question

asked Mar 23, 2019 1.2k views

2 Answers

Jacoblambert · Answer 1 · 2019-03-25T17:19:30+0000

let x be the name for that number, write the inequalities:

$0.5x+3>0 \\0.5x>-3\\x>-6\\\mbox{OR}\\0.5x+3\leq -3\\0.5x\leq -6\\x\leq -12\\$

This number x can be anything, except anything in the interval (-12, 6] (excluding -12, and including 6). Lmk if you have questions.

Luca Marzi · Answer 2 · 2019-03-30T18:32:49+0000

Answer:

The solutions of given inequalities are
x>-6 and
$x\leq -12$ respectively.

Explanation:

It is given that one half a number increased by three is greater than zero or less than or equal to negative three.

Let x be the unknown number.

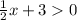(1)/(2)x+3>0 or
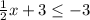
$(1)/(2)x+3\leq -3$

On solving first inequality,

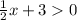
(1)/(2)x+3>0

Subtract 3 from both sides.

(1)/(2)x>-3

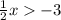
Multiply both sides by 2.

x>-6

On solving second inequality,

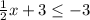
$(1)/(2)x+3\leq -3$

Subtract 3 from both sides.

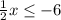
$(1)/(2)x\leq -6$

Multiply both sides by 2.

$x\leq -12$

Therefore, the solutions of given inequalities are
x>-6 and
$x\leq -12$ respectively.