Answer:
Maximum revenue = 1000 thousands of dollars.
Step-by-step explanation:
We have the revenue equation
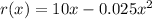 , where x is measured in thousands of toys produced, and r(x) is measured in thousands of dollars.
, where x is measured in thousands of toys produced, and r(x) is measured in thousands of dollars.
At maximum revenue the derivative of equation is zero
So,
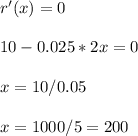
So maximum revenue is when 200 thousands of toys are produced.
Maximum revenue,
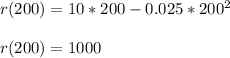
Maximum revenue = 1000 thousands of dollars.