QUESTION 1
The price of a bottle of water is $1.50 dollars
If
 units are sold, the total cost is
units are sold, the total cost is
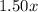
We can write the total cost as a function of the unit price.
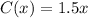
To graph this function we can choose at least two points.
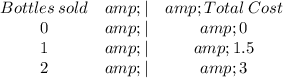
We now plot these points and use it to draw our graph. See attachment.
QUESTION
At a point on the graph we can determine that, for every 6 amount of salt added, 18 amount of tomatoes is also added.
So the unit rate of the number of tomatoes to the number of salt added is
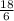
We express the denominator as one to get the unit rate, so we divide both the numerator and denominator by 3.
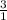
Therefore the unit rate of tomatoes to salt is 3:1 or simply 3.
QUESTION 3
We calculate the rate of relationship B and compare to the rate of the given options,
The graph passes through,
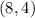
and
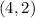
So the rate of this graph is the same as the slope,
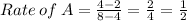
The rate of B is greater than
 . So we select all the relationships that has a slope greater than
. So we select all the relationships that has a slope greater than
 .
.
These are;
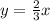 ,
,
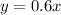 and
and
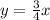
QUESTION 4
We again find the rate of relationship B and compare it to the rates in the given options. We choose any two points from the table and use it to find the rate of B.
Let us use,
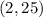 and
and


Since relationship A is greater, we choose all the relationship that has a slope more than 12.5.
These are,
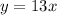 and
and
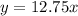
QUESTION 5
Again we find the rate of printer B and compare it to printer A.
Let us use the points,
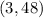 and
and
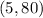
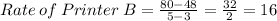
Hence Printer A prints 14 words per minute, while printer B prints 16 words per minute.
Therefore printer B prints faster than printer A.