Question

Given that (2,8) is on the graph of f(x),find the corresponding point for the function -3/4 f(x)

Answer 1

The corresponding point on the graph of the function -3/4 f(x) when given that (2,8) is on the graph of f(x) is (2, -6), achieved by scaling the y-value by a factor of -3/4.

Step-by-step explanation:

The question involves finding the corresponding point on the graph of the function -3/4 f(x) given that (2,8) is on the graph of f(x). Since f(x) at x=2 is 8, for the new function -3/4 f(x) at the same x value, the y value will be scaled by a factor of -3/4.

Therefore, we calculate the new y value by multiplying 8 by -3/4, which results in -6. The corresponding point on the graph of the function -3/4 f(x) when x=2 is therefore (2, -6).

Answer 2

Corresponding point to (2,8) along -3/4 f(x) = ( -1.5 , -6 )

Step-by-step explanation:

When a graph f(x) is multiplied by a factor k, the points on the graph also changes by (kx, ky).

Here it is given that (2,8) is on the graph of f(x)

Now we need to find corresponding point to (2,8) along -3/4 f(x)

x - coordinate =
`(-3*2)/(4) =-1.5`

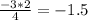
y - coordinate =
`(-3*8)/(4) =-6`

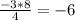
So corresponding point to (2,8) along -3/4 f(x) = ( -1.5 , -6 )