Question

a play is performed for a crowd of 400 people.adult ticket cost $22 each, student tickets cost $15 each,and tickets for children cost $13.50 each.the revenue for the concert is $7840.there are 40 more children at the concert than students.how many of each type of ticket are sold?

Answer 1

For this case we have the following variables:

A: Represents the number of adults

S: Represents the number of students

C: Represents the number of children

For the income we have:

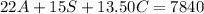`22A + 15S + 13.50C = 7840` -----> (1)

Regarding the number of people at the concert we have:

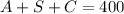`A + S + C = 400` -----> (2)

If there are 40 more children than students, we have:

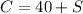`C = 40 + S` -----> (3)

We substitute C in the second equation and it remains:

`A + S + 40 + S = 400`

`A + 40 + 2S = 400`

`A + 2S = 400-40`

`A + 2S = 360`

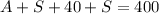
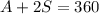
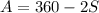`A = 360-2S` --------> (4)

Substituting (3) and (4) in (1) we have:

`22 (360-2S) + 15S + 13.50 (40 + S) = 7840`

`7920-44S + 15S + 540 + 13.50S = 7840`

`7920-7840 + 540 = 44S-15S-13.50S`

`620 = 15.50S`

`S = (620)/(15.50)`

`S = 40`

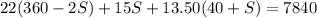
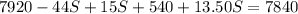
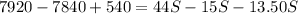
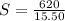
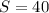
So, there are 40 children.

Substituting S in (3) and obtaining C:

`C = 40 + S`

`C = 40 + 40`

`C = 80`

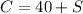
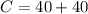
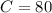
Substituting S in (4):

`A = 360-2 (40)\\A = 360-80\\A = 280`

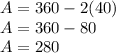
Thus, 280 tickets of adults, 80 of children and 40 of students were sold.

Answer:

280 tickets for adults, 80 for children and 40 for students