Question

In a coastal town, the humidity, measured in grams of water per kilogram of air, increases by 43% for every 1 degree celsius increase in temperature. A scientist observes that the humidity on monday morning is 5.75 grams per kilogram. He wants to know how much the temperature must increase for the humidity to reach at least 49.17 grams per kilogram.

Answer 1

In order to increase humidity by 49.17 grams per kilogram, the increase in temperature will be 18 degree Celsius approx.

Explanation:

Initial humidity = 5.75 g/kg

Final humidity= 49.17 g/kg

Percentage increase in humidity= (49.17-5.75)/5.75 *100= 755.13

Increase in temperature= 755.13/43 = 17.56

Increase in temperature = 18 degree Celsius approx.

Answer 2

The temperature must increase by more than 6 degree Celsius for the humidity to reach at least 49.17 grams per kilogram

Explanation:

Given : Humidity on Monday morning = 5.75 grams per kilogram

To Find: He wants to know how much the temperature must increase for the humidity to reach at least 49.17 grams per kilogram.

Solution :

Let T be the increase in temperature, in degrees celsius, from monday morning.

According to question

The humidity measured in grams of water per kilogram of air, increases by 43% for every 1 degree Celsius increase in temperature.

So. Rate of increment is 43%

So, it becomes :
`100+43=143\% =1.43`

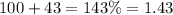
43% increases for every 1 degree celsius.

So, for T the increase is 1.43*T

So,
`1.43T\geq (49.17)/(5.75)`

`1.43T\geq 8.55`

`T\geq (8.55)/(1.43)`

`T\geq 5.97`

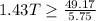
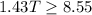
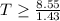
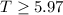
Thus the temperature must increase by more than 6 degree Celsius for the humidity to reach at least 49.17 grams per kilogram