Question

A cylindrical block of aluminum has a radius 2.5 cm and

length 20 meters. The center of the cylinder has 20 mm of
aluminum removed radially
(A) What is the resistance of this block of material?
(B) What temperature would the aluminum have to be at to have the
same resisttivity as tungsten of the same size and length?
C) How much power is dissipated when 30 amps of is passed through
the cylindrical block using the resistance from part A.

Answer 1

(A) The resistance of the block of aluminum is given by:

`R=(\rho L)/(A)`

where

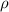`\rho` is the aluminum resistivity

L is the length of the cylinder

A is the cross-sectional area of the cylinder

We already know the aluminum resistivity (
`\rho=2.65 \cdot 10^(-8) \Omega m`) and the length of the cylinder (L=20 m), so we must find the cross-sectional area A, which is given by the difference between the area of the larger cylinder and the area of the radial hole:

`A=\pi (R^2 -r^2)`

where
`R=2.5 cm=0.025 m` and
`r=20 mm=0.02 m` (assuming that the 20 mm removed radially refers to the radius of the hole).

Therefore, the cross-sectional area is

`A=\pi ((0.025 m)^2-(0.020 m)^2)=7.06 \cdot 10^(-4) m^2`

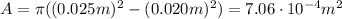
Substituting into the initial formula of the resistance, we find:

`R=(\rho L)/(A)=((2.65 \cdot 10^(-8) \Omega m)(20 m))/(7.06 \cdot 10^(-4) m^2)=7.51 \cdot 10^(-4) \Omega`

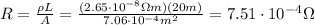
(B) The resistivity of the tungsten is
`\rho=5.6 \cdot 10^(-8) \Omega m`, so a cylinder of tungsten of the same size would have a resistance of

`R=(\rho L)/(A)=((5.6 \cdot 10^(-8) \Omega m)(20 m))/(7.06 \cdot 10^(-4) m^2)= 1.59 \cdot 10^(-3) \Omega`

The behaviour of the resistance versus temperature is given by:

`R=R_0 (1 + \alpha \Delta T)`

where
`\alpha` is a coefficient that for aluminum is equal to
`\alpha = 0.004308`, R0 is the resistance of the piece of aluminum we found at point (A), and R is the resistance of the tungsten. Re-arranging the formula and substituting, we find

`\Delta T = (R/R_0 -1)/(\alpha)=((1.59 \cdot 10^(-3) \Omega)/(7.51 \cdot 10^(-4) \Omega)-1)/(0.004308)=259.3^(\circ)C`

So, the temperature must increase by 259.3 degrees.

(C) The power dissipated is given by:

`P=I^2 R`

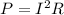
where I=30 A is the current. Substituting the numbers into the formula, we find

`P=(30 A)^2 (7.51 \cdot 10^(-4) \Omega)=0.68 W`