Question

Determine whether AB ← → and CD ← → − are parallel, perpendicular, or neither. A(2, 8), B(−1, −2), C(3, 7), D(0, −3)

Answer 1

AB and CD are parallel to each other.

Explanation:

We have to check whether the line segment AB and CD are parallel, perpendicular or nothing,

The coordinates of A, B, C , D are:

A(2, 8), B(−1, −2), C(3, 7), D(0, −3)

We calculate the slope of line segment AB and CD.

Formula:

`\text{Slope} = \displaystyle(y_2-y_1)/(x_2-x_1)\\\text{where }(x_1,y_1), (x_2, y_2)\text{ are the coordinates of the endpoints of line segment}`

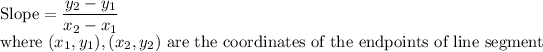
Putting the values, we get,

Slope of Line segment of AB =

`\displaystyle(-2-8)/(-1-2) = (-10)/(-3)=(10)/(3)`

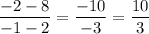
Slope of Line segment of CD =

`\displaystyle(-3-7)/(0-3) = (-10)/(-3)=(10)/(3)`

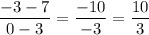
Thus,

Slope of Line segment of AB = Slope of Line segment of CD

Hence, the two line segments AB and CD are parallel to each other.

Answer 2

A plot of the points quickly reveals the vectors to be parallel.