Answer:
The solutions of the given equation are
 and
and
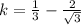 .
.
Step-by-step explanation:
The given equation is

Divide both sides by 9.
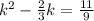
If an expression is
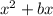 then we need to add
then we need to add
 in the expression to make it perfect square.
in the expression to make it perfect square.
In the above equation
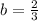 , so add
, so add
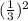 on both the sides.
on both the sides.

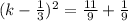
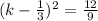
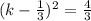
Taking square root both the sides.
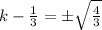
Add 1/3 on both the sides.

Therefore the solutions of the given equation are
 and
and
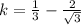 .
.