Question

What is the solution of 3x+8/x-4 >;= 0

Answer 1

A. x ≤ −8/3 or x>4

Explanation:

Edge 2020 answer is A

Answer 2

The division between two numbers is positive if and only if they have the same sign. So, this fraction is positive if numerator and denominator are either both positive or both negative.

For this reason, you want to study the sign of numerator and denominator separately first.

As for the numerator, you have

`3x+8 \geq 0 \iff 3x \geq -8 \iff x \geq -(8)/(3)`

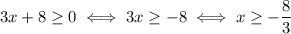
Similarly, for the denominator you have

`x-4 > 0 \iff x > 4`

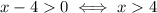
(note that we used strict inequality for the denominator, since it can't be zero).

So, the sign of the fraction works like this:

• If
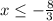  `x \leq -(8)/(3)` both numerator and denominator are negative (or, at most, the numerator is zero if
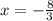  `x = -(8)/(3)`), so the ratio is greater than or equal to zero.
• If
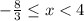  `-(8)/(3) \leq x < 4` the numerator is positive and the denominator is negative (or, at most, the numerator is zero if
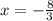  `x = -(8)/(3)`), so the ratio is less than or equal to zero.
• If
  `x >4` both numerator and denominator are positive, so the ratio is greater than or equal to zero.