Answer:
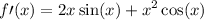
Explanation:
We are given a function:
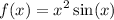
Notice that there are two functions multiplying each other. Recall the product rules.
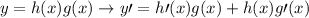
You can let x^2 = h(x), sin(x) = g(x) or sin(x) = h(x), x^2 = g(x) as your desire but I’ll let h(x) = x^2 and g(x) = sin(x).
Therefore, from a function:
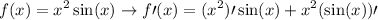
Recall the power rules and differentiation of sine.

Therefore, from differentiating function,
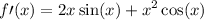
And we are done!