Question

Given a population with mu = 64 and sigma = 25, if one draws samples of size l55, the sample means will have a distribution which is approximately normal with:

Answer 1

Solution: We are given a population follows a normal distribution with Mean
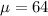`\mu=64` and standard deviation
`\sigma=25`

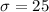
According to central limit theorem, if we have a population with mean
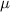`\mu` and standard deviation
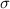`\sigma` and take large samples from the population, then the distribution of samples means will be approximately normally distributed with mean and standard deviation given below:

`\mu_{\bar{x}}=\mu`

`\sigma_{\bar{x}}=(\sigma)/(√(n))`

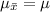
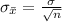
Therefore, for the given example the distribution of sample means will be approximately normal with mean and standard deviation given below:

`\mu_{\bar{x}}=\mu=64`

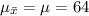
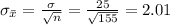`\sigma_{\bar{x}}=(\sigma)/(√(n))=(25)/(√(155))=2.01` rounded to 2 decimal places.