Answer:
Option B. 0.6 seconds
Explanation:
As given in the table at time t = 0 the maximum height of the penny is 2 meters.
In simpler way we can say the penny has been throw from a height of 2 meters.
Now this process can be represented by the equation of motion
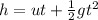
For free fall u = 0
So
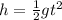
where h = 2 meters
and g = 9.81 m/sec²
By putting these values in the equation
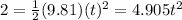

t = √0.4077 = 0.6385 seconds
or t = 0.6 seconds
Answer is option B. 0.6 seconds