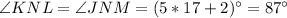Question

What is m∠KNL? Enter your answer in the box. ° A horizontal line segment M K intersects with line segment J L at their midpoint N. The upward-left and downward-right angles formed by these lines are obtuse. The upward-left obtuse angle is labeled left parenthesis 5 x plus 2 right parenthesis degrees. The downward-right acute angle is labeled left square bracket 3 left parenthesis x plus 14 right parenthesis right square bracket degrees.

Answer 1

The measure of
`\angle KNL = 87\°`

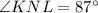
Explanation:

According to the below diagram, horizontal line segment
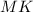`MK` intersects with
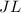`JL` at their midpoint
`N`.

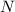So,
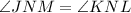`\angle JNM = \angle KNL` (As they are vertical angles)

Given that,
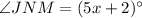`\angle JNM = (5x+2)\°` and
`\angle MNL = [3(x+14)]\°`

As they are adjacent angles and points
`J, N` and
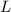`L` are co-linear, so
`\angle JNM +\angle MNL = 180\°`

That means.......

`(5x+2)+3(x+14)=180\\ \\ 5x+2+3x+42=180\\ \\ 8x+44=180\\ \\ 8x=180-44=136\\ \\ x=(136)/(8)=17`

Thus,
`\angle KNL =\angle JNM= (5*17+2)\° = 87\°`