Answer:
The vertices of parallelogram ESTA are E (10, 0), S (14, 3), T (6, 9), and A (2, 6).
As we know ,Area of parallelogram = Base × Height
As base can be determined by using distance formula because coordinates of the vertices are given.
Now to determine perpendicular, we will find the equation of line through E and perpendicular to ST.
Equation of line through S(14,3) and T(6,9) is
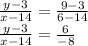

⇒4y + 3x =12+42
⇒ 3 x + 4 y=54
Equation of line perpendicular to ST and passing through E(10,0) is
4 x-3 y + k=0
Put x=10, y=0 in above equation is
4×10 -3×0 +k=0
⇒ k= -40
The Equation of line through point E and perpendicular to ST is
4 x-3 y -40=0