We are given
First expression:
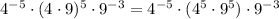
We used property
power rule-II:
Product to power distribute to each base
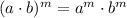
Second expression:
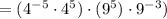
we used property
associative property of multiplication:
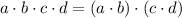
Third expression:
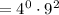
we used property
Product Rule:
Same base add exponents
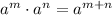
Fourth expression:
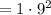
we used property
Zero Exponent :
Anything to the zero power (except 0) is one
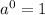
Fifth expression:

we used property
Multiplicative identity:

Sixth expression:
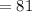
We used simplification