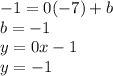Answer:
1.
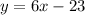
2.
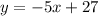
3.

4.
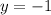
Step-by-step explanation:
- The equation of the line is:

Where
 is the slope and
is the slope and
 is the y-intercept.
is the y-intercept.
- You have to find the y-intercept
 , so, you must substitute the given point and the slope into each equation and solve for
, so, you must substitute the given point and the slope into each equation and solve for
 , and then you must rewrite the equation of the line with the slope and the y-intercept calculated:
, and then you must rewrite the equation of the line with the slope and the y-intercept calculated:
1.
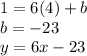
2.

3.
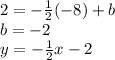
4.