Question

HELP PLEASE??

Drag and drop the answers into the boxes to correctly complete the statement.

A sequence of transformations that maps △ABC to △A′B′C′ is a _____________ followed by a _____________.

- reflection across the y-axis
- rotation of 180° about the origin
- translation 2 units right
- rotation of 90° counterclockwise about the origin

Answer 1

(1) Rotation of 90° counterclockwise about the origin (2) Translation 2 units right .

Explanation:

We have been asked to determine a sequence of transformations that maps △ABC to △A′B′C'.

To map △ABC to △A′B′C′ first of all we have to rotate △ABC 90° counterclockwise (positive rotation) about the origin. After rotating △ABC 90° our new coordinates will be A(-3,-4), B(-3,-1) and C (-1,-2).

We can see that distance between A and origin is
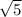`√(5)` and distance between A' and origin is also
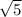`√(5)`. Distance between A and A' is
`√(10)`, which forms a right triangle.

To map △ABC to △A′B′C′ completely we will translate our △A′B′C' 2 units right. After translation our coordinates will be A'(-1,-4), B'(-1,-1) and C'(1,-2).

Therefore, a sequence of transformations that maps △ABC to △A′B′C′ is (1) rotation of 90° counterclockwise about the origin and (2) translation 2 units right.