Answer:
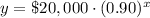
Explanation:
We have been given that Kelly bought a new car for $20,000. The car depreciates at a rate of 10% per year. We are asked to write an equation to model the car's value.
Since car's value depreciates by 10% per year, so value of car is depreciating exponentially.
We know that an exponential decay function is in form
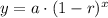 , where
, where
a = Initial value,
r = Decay rate in decimal form.
Let us convert 10% into decimal form as:

Upon substituting our given values in decay formula, we will get:
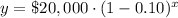
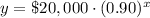
Therefore, our required equation would be
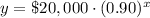 .
.