Speed up to Mississippi Rive = 12 mph
Speed back to original port = 15 mph
Total time taken by the riverboat = 7.5 hours
Total distance covered = ?
We know that the distance covered each side would be the same.
So Distance up to Mississippi Rive = Distance back to original port
⇒
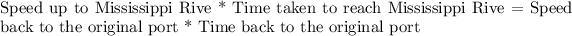 .......... (i)
.......... (i)
Let Time taken to reach Mississippi Rive = x .............. (ii)
⇒ Time taken to reach back to original port = 7.5 - x .......... (iii)
Substituting (ii) and (iii) in (i)
⇒
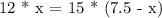
⇒
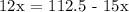
⇒

⇒
/(27)](https://img.qammunity.org/2019/formulas/mathematics/middle-school/wb3dajnfoypekgtu1oy0981sinoy41r8vo.png) [/tex]
[/tex]
Using value of x to determine distance traveled:
Distance Traveled up to Mississippi Rive =
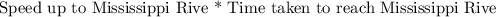
⇒ Distance Traveled up to Mississippi Rive =

⇒ Distance Traveled up to Mississippi Rive = 50 miles
Hence, the riverboat traveled total of 50 + 50 = 100 miles