Question

△klm, lm=20 sqrt 3 m∠k=105°, m∠m=30° find: kl and km

Answer 1

KL =
`(20√(6))/(1+√(3))` = 17.93

MK =
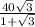`(40√(3))/(1+√(3))` = 25.36

Step-by-step explanation:

According to the Law of Sines:

`(a)/(sinA)=(b)/(sinB)= (c)/(sinC)`

where:

A, B, and C are angles

a, b, and c are the sides opposite to the angles

First of all, let's find m∠L: the sum of the angles of a triangle is 180°, therefore

m∠K + m∠L + m∠M = 180°

m∠L = 180° - m∠K - m∠M

m∠L = 180° - 105° - 30°

m∠L = 45°

Now, we can apply the Law of Sines to our case (see picture attached):

`(LM)/(sinK)=(MK)/(sinL)=(KL)/(sinM)`

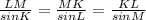
Let's solve one side at the time:

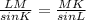
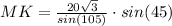
`(LM)/(sinK)=(MK)/(sinL)`

`(20√(3))/(sin(105))=(MK)/(sin(45))`

`MK = (20√(3) )/(sin(105)) \cdot sin(45)`

MK =
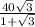`(40√(3) )/(1+√(3) )` = 25.36

Similarily:

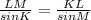
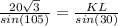
`(LM)/(sinK)=(KL)/(sinM)`

`(20√(3))/(sin(105))=(KL)/(sin(30))`

`KL = (20√(3) )/(sin(105)) \cdot sin(30)`

KL =
`(20√(6))/(1+√(3))` = 17.93