Answer:
Yes, Josh's parent's house in Texas is the midpoint between South Carolina and California
Explanation:
Let S represents South carolina C represents California and T represents Texas,
Given,
ST = 12x - 106,
TC = 5x + 685,
Also, SC = 2500 miles,
Suppose T is the midpoint of S and C,
⇒ ST = TC
12x - 106 = 5x + 685
7x = 791
⇒ x =
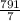 = 113
= 113
So, ST =
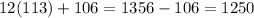
Now,

⇒ 2 ST = SC
Hence, T is the midpoint of S and C.