we have
1) The line passes through
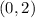
2) The slope is equal to

we know that
If a point lies on the graph of the line, then the point must satisfy the equation of the line
Step 1
Find the equation of the line
we know that
the equation of the line in point-slope form is equal to
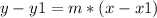
we have
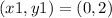
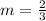
substitute in the equation
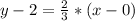
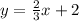
we will proceed to verify each of the points to determine the solution of the problem
If a point lies on the graph of the line, then the point must satisfy the equation of the line and the equation will be true for the point
Step 2
Point
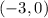
Substitute the value of x and y in the equation of the line
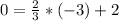

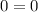 -------> is true
-------> is true
therefore
the point
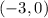 lies on the line
lies on the line
Step 3
Point
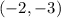
Substitute the value of x and y in the equation of the line
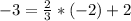
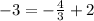
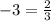 ------> is false
------> is false
therefore
the point
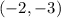 not lies on the line
not lies on the line
Step 4
Point
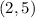
Substitute the value of x and y in the equation of the line
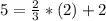
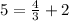
 ------> is false
------> is false
therefore
the point
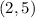 not lies on the line
not lies on the line
Step 5
Point
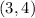
Substitute the value of x and y in the equation of the line
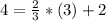
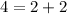
 ------> is True
------> is True
therefore
the point
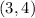 lies on the line
lies on the line
Step 6
Point
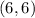
Substitute the value of x and y in the equation of the line

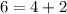
 ------> is True
------> is True
therefore
the point
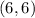 lies on the line
lies on the line
therefore
the answer is
Vera could use the points
the point
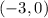
the point
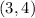
the point
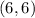
using a graphing tool
see the attached figure to better understand the problem