Question

1. Find the value of x. The diagram is not to scale. Lines F and G are parallel.

A. 10
B. 11
C. 12
D. -11

2. Find the value of x for which I is parallel to M. The diagram is not to scale.

A. 28
B. 56
C. 84
D. 152

3. Use the diagram to answer the question. Fill in the blank for the letter given with the missing reason in the flow proof.

Answer 1

Part 1:

we have to find the value of x

we have been given the lines f and g are parallel

Interior angles on the same side are supplementary.

Hence, the two given angles will be equal to 180

`5x^(\circ)+9x^(\circ)+26^(\circ)=180^(\circ)`

`14x^(\circ)=180^(\circ)-26^(\circ)`

`14x^(\circ)=154^(\circ)`

`x=11^(\circ)`

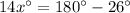
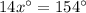
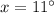
Therefore, Option B is correct.

Part 2:

We have to find x

l and m are parallel

Two parallel lines are cut by Transverse line

And angles on the opposite sides that are alternate interior angles are equal

Hence,
`x=28^(\circ)`

Therefore, option A is correct.

Part 3:

a. m∠5=
`40^(\circ)`

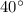
and m∠2=
`140^(\circ)`

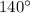
b. m∠5+m∠2=
`40^(\circ)+140^(\circ)=180^(\circ)`

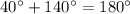
c.∠5 and ∠2 are supplementary because they are
`180^(\circ)`

d.∠5 and ∠2 are same side interior angles since, they are
`180^(\circ)`

e. a || b since, a is
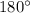`180^(\circ)` and b is also
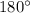`180^(\circ)`.

Answer 2

(1)

we are given that

f and g are parallel lines

so, sum of both angles must be 180

`5x+9x+26=180`

now, we can solve for x

`14x+26=180`

Subtract both sides by 26

`14x+26-26=180-26`

`14x=154`

`x=11`...........Answer

(2)

we are given that

l and m are parallel lines

so, alternate angles must be equal

so, we get

`x=28`...........Answer