Question

Find the distance between points (30, 24) and (1, 21). Round to the nearest hundredth if necessary.

Answer 1

Answer: The distance between (30,24) and (1,21) is about 29.15 units

Step-by-step explanation: It may help to sketch the points on a graph to see where they are, and get an idea of the shape of the right triangle that would be formed. The distance between the points is the hypotenuse of the triangle, so the Pythagorean Theorem will solve for the distance.

a² + b² = c²

24-21= 3 30-1=29 These are the lengths of sides a & b

3² = 9 29²= 841 9 + 841 = 850 The square root of 850 will be the length of side c, the hypotenuse.

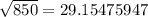`√(850) = 29.15475947` Round to the nearest hundredth: 29.15