Given:-
 ;
;
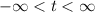 , where a is any positive real number.
, where a is any positive real number.
Consider the helix parabolic equation :

now, take the derivatives we get;
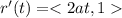
As, we know that two vectors are orthogonal if their dot product is zero.
Here,
 are orthogonal i.e,
are orthogonal i.e,
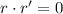
Therefore, we have ,

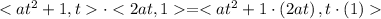
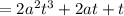
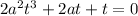
take t common in above equation we get,
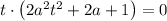
⇒
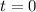 or
or
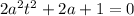
To find the solution for t;
take
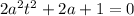
The number
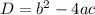 determined from the coefficients of the equation
determined from the coefficients of the equation
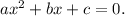
The determinant
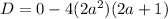
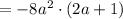
Since, for any positive value of a determinant is negative.
Therefore, there is no solution.
The only solution, we have t=0.
Hence, we have only one points on the parabola
 i.e <1,0>
i.e <1,0>