Question

The length of a rectangle is 24 units. Can the perimeter P of the rectangle be 60 units when its width w is 11 units? No, because P ≠ 2(24) + 2w No, because P = 2(24) + 2w Yes, because P ≠ 2(24) + 2w Yes, because P = 2(24) + 2w

Answer 1

P = 2L + 2W

P = 2x24 + 2x11

P = 70

So your answer is No, because P ≠ 2(24) + 2w

Answer 2

No, Because P ≠ 2(24) + 2w

A is correct.

Explanation:

The length of a rectangle is 24 units.

The perimeter of rectangle is P = 60 unit

If width of rectangle is 11 units (w)

Formula:

Perimeter of rectangle (P) = 2(L+W)

where, L=24 and W=11

P = 2(24+11)

`P=70`

where, P=60

`60\\eq 70`

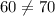
Therefore, P ≠ 2(24) + 2w

No, The perimeter can not be 60 unit of this rectangle.