Answer:
31.38 s (2 d.p.)
Step-by-step explanation:
Constant Acceleration Equations (SUVAT)
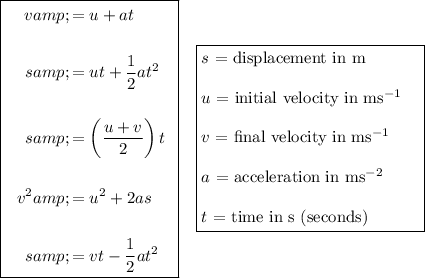
When using SUVAT, assume the object is modeled as a particle and that acceleration is constant.
-----------------------------------------------------------------------------
To find the minimum time in which an elevator can travel the 373 m from the ground floor to the observatory floor, we to accelerate to the maximum allowed velocity as quickly as possible, maintain the maximum velocity for as long as possible, and then decelerate for the shortest period of time in order for the elevator to come to a stop after 373 m.
Therefore, the travel time from the start of the elevator trip to the end will have three phases:
- Acceleration
- Constant velocity
- Deceleration
Phase 1 - Acceleration
Given information:
The time it takes to accelerate from rest to the maximum allowed velocity, where the elevator accelerates at the maximum allowed acceleration:
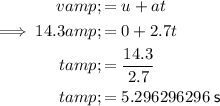
The distance covered in this time is:
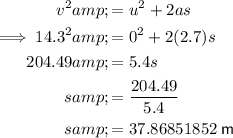
Phase 3 - Deceleration
Given information:
When the elevator is decelerating from the maximum velocity to being at rest, it will take the same amount of time and cover the same distance as its acceleration phase:
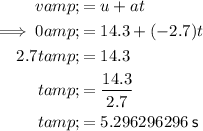
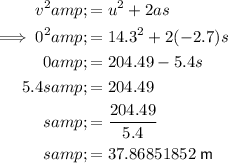
Phase 2 - constant velocity
The total distance the elevator travels is 373 m.
Therefore, the distance it travels during phase 2 when it is traveling at a constant (maximum) velocity is the total distance less the distance traveled in phases 1 and 3:

Given information:
Therefore, the time is takes to travel this distance when it is traveling at a constant (maximum) velocity is:

Total minimum time
Therefore, the minimum time is the sum of the time calculated for the three phases:
