Question

Select all irrational numbers.

14√

24√

34√

44√

54√

Answer 1

1,2,3? hope it helped

Answer 2

All of the above

Explanation:

We have to find the irrational numbers.

Irrational number: The number which can not be written as
`(p)/(q)` form, where p and q are integers and
`q\\eq 0` then, the number is called irrational number.

a.
`√(14)`

`√(2* 7)`

`√(2)* √(7)`

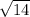
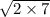
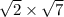
We know that
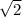`√(2)` and
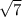`√(7)` are irrational numbers and product of two different irrational numbers is irrational number.

Hence, it is irrational number.

b.
`√(24)`

`√(2* 2* 2 * 3)`

`2√(6)`

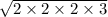
We know that
`\sqrt6` is a irrational number and 2 is rational number.The product of irrational number and rational number is irrational number.

Therefore,
`√(24)` is irrational number.

c.
`√(34)`

`√(2)* √(17)`

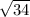
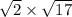
We know that
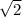`√(2)` and
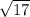`√(17)` are irrational numbers and product of two different irrational numbers is irrational number.

Hence, it is irrational number.

d.
`√(44)`

`√(2* 2* 11)`

`2√(11)`

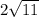
We know that
`√(11)` is a irrational number and 2 is rational number.The product of irrational number and rational number is irrational number.

Hence, it is irrational number.

e.
`√(54)`

`√(2* 3* 3* 3)`

`3√(6)`

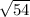
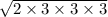
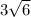
We know that
`\sqrt6` is a irrational number and 3 is rational number.The product of irrational number and rational number is irrational number.

Hence, it is irrational number.