The ball's horizontal velocity is constant throughout its trajectory, with a magnitude of
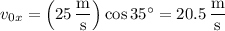
If the ball has landed before 1 second has passed, then its velocity will be zero, so we should check if that's the case before we wrap up. The ball's vertical position in the air is given by
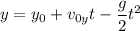
where we can assume
 is the ball's initial position, and
is the ball's initial position, and
 is the acceleration due to gravity. Then the time it takes for the ball to reach the ground after being kicked is
is the acceleration due to gravity. Then the time it takes for the ball to reach the ground after being kicked is

So it takes the ball 2.93 seconds to reach the ground, so that after 1 second in particular, the ball's horizontal velocity is still 20.5 m/s.